1. What is the Ideal Gas Pressure Calculator?

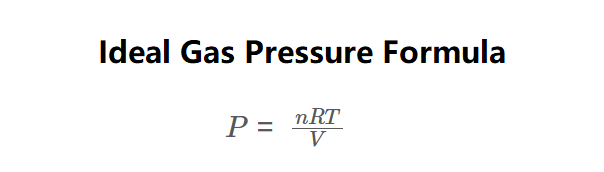
Definition: This calculator computes the pressure (\( P \)) of an ideal gas using the ideal gas law, as shown in the formula image above. Pressure is calculated via:

\( P = \frac{nRT}{V} \)

Users input volume (\( V \)), amount of substance (\( n \)), and temperature (\( T \)), with support for multiple units. Results are displayed with 5 decimal places for precision, in units such as Pa, kPa, atm, bar, psi, or mmHg.[](https://www.omnicalculator.com/physics/ideal-gas-pressure)

Purpose: Essential for thermodynamics, chemical engineering, and physics to determine gas pressure in systems like reactors, engines, or atmospheric studies.

2. How Does the Calculator Work?

The calculator uses the ideal gas law (\( PV = nRT \)), rearranged to solve for pressure:

\( P = \frac{nRT}{V} \)

Where:

• \( P \): Pressure (Pa, kPa, atm, bar, psi, mmHg);
• \( V \): Volume (m³, cm³, L, cu ft);
• \( n \): Amount of substance (mol);
• \( T \): Temperature (K, °C, °F);
• \( R \): Universal gas constant, default 8.31446261815324 J/(mol·K).

Common Gases and Molecular Masses (for Reference):

Gas	Molecular Mass (g/mol)
Air	28.96
Nitrogen	28.02
Oxygen	32.00
Carbon Dioxide	44.01
Methane	16.04

Steps:

• Enter volume with units (m³, cm³, L, cu ft).
• Enter amount of substance in moles.
• Enter temperature with units (K, °C, °F).
• Enter the universal gas constant (\( R \)), defaulting to 8.31446261815324 J/(mol·K).
• Submit to calculate pressure.
• Change the output unit (Pa, kPa, atm, bar, psi, mmHg) to convert the result instantly.
• Results are formatted to 5 decimal places, with scientific notation for values less than 0.001.

3. Importance of Ideal Gas Pressure Calculation

Gas pressure calculations are critical for:

• Chemical Engineering: Designing reactors and gas storage systems.
• Aerodynamics: Analyzing gas behavior in high-altitude or propulsion systems.
• Environmental Science: Studying atmospheric pressure variations.

4. Using the Calculator

Example 1: Calculate the pressure of 1 mol of gas in 1 m³ at 25°C, with \( R = 8.31446261815324 \, \text{J/(mol·K)} \), output in Pa and atm:

• Volume: \( V = 1 \, \text{m³} \)
• Amount of Substance: \( n = 1 \, \text{mol} \)
• Temperature: \( T = 25 \, \text{°C} = 298.15 \, \text{K} \)
• Calculation: \( P = \frac{1 \times 8.31446261815324 \times 298.15}{1} \approx 2478.63300 \, \text{Pa} \)
• Unit conversion: \( 2478.63300 \div 101325 \approx 0.02446 \, \text{atm} \)

Results:

• Pressure: 2478.63300 Pa
• Pressure (converted): 0.02446 atm

Example 2: Calculate the pressure of 0.5 mol of gas in 500 cm³ at 77°F, output in kPa and mmHg:

• Volume: \( V = 500 \, \text{cm³} = 0.0005 \, \text{m³} \)
• Amount of Substance: \( n = 0.5 \, \text{mol} \)
• Temperature: \( T = 77 \, \text{°F} = (77 - 32) \times \frac{5}{9} + 273.15 = 298.15 \, \text{K} \)
• Calculation: \( P = \frac{0.5 \times 8.31446261815324 \times 298.15}{0.0005} \approx 2478633.00000 \, \text{Pa} \)
• Unit conversion:
  • kPa: \( 2478633.00000 \div 1000 \approx 2478.63300 \, \text{kPa} \)
  • mmHg: \( 2478633.00000 \div 133.322368 \approx 18592.05843 \, \text{mmHg} \)

Results:

• Pressure: 2478.63300 kPa
• Pressure (converted): 18592.05843 mmHg

5. Frequently Asked Questions (FAQ)

Q: What is the ideal gas law?
A: The ideal gas law (\( PV = nRT \)) relates pressure, volume, amount of substance, and temperature for an ideal gas, assuming no intermolecular forces and elastic collisions.

Q: Why use Kelvin for temperature?
A: Kelvin is an absolute scale, ensuring positive temperatures for accurate calculations.

Q: Can this calculator be used for real gases?
A: Yes, for low pressures and high temperatures where real gases approximate ideal behavior. For high pressures, consider the van der Waals equation.

Q: What is the universal gas constant?
A: The universal gas constant (\( R \)) is 8.31446261815324 J/(mol·K), relating energy to temperature and moles in the ideal gas law.

Q: How do I convert between pressure units?
A: Use the output unit dropdown to instantly convert pressure to Pa, kPa, atm, bar, psi, or mmHg.

Ideal Gas Pressure Calculator © - All Rights Reserved 2025