1. What is the Gas Density Calculator?

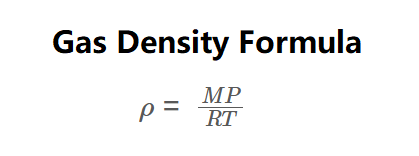
Definition: This calculator computes the density (\( \rho \)) of an ideal gas, defined as mass per unit volume (\( \rho = \frac{m}{V} \)), using the ideal gas law. As shown in the formula image above, density is calculated via:

\( \rho = \frac{MP}{RT} \)

Users input pressure (\( P \)), temperature (\( T \)), and molecular mass (\( M \)), with support for multiple units and predefined gases. Results are displayed with 5 decimal places for precision.

Purpose: Essential for applications in thermodynamics, aerodynamics, and chemical engineering to analyze gas behavior, such as in aircraft design or pipeline engineering.

2. How Does the Calculator Work?

The calculator derives gas density from the ideal gas law (\( PV = nRT \)), rearranging to:

\( \rho = \frac{MP}{RT} \)

Where:

• \( \rho \): Density (kg/m³, lb/cu ft, lb/cu yd, g/cm³, kg/cm³, mg/cm³, g/m³, g/dm³, g/L);
• \( M \): Molecular mass (kg/mol);
• \( P \): Pressure (Pa, kPa, atm, bar, psi, mmHg);
• \( R \): Universal gas constant, default 8.31446261815324 J/(mol·K);
• \( T \): Temperature (K, °C, °F).

Available Gases and Molecular Masses:

Gas	Molecular Mass (g/mol)
Air	28.96
Nitrogen	28.02
Oxygen	32.00
Carbon Dioxide	44.01
Methane	16.04
Custom	User-defined

Steps:

• Select a gas or choose "Custom" to enter molecular mass.
• Enter molecular mass in g/mol or kg/mol if custom.
• Enter pressure with units (Pa, kPa, atm, bar, psi, mmHg).
• Enter temperature with units (K, °C, °F).
• Enter the universal gas constant (\( R \)), defaulting to 8.31446261815324 J/(mol·K).
• Submit to calculate density.
• Change the output unit (kg/m³, lb/cu ft, lb/cu yd, g/cm³, kg/cm³, mg/cm³, g/m³, g/dm³, g/L) to convert the result instantly.
• Results are formatted to 5 decimal places, with scientific notation for values less than 0.001.

3. Importance of Gas Density Calculation

Gas density is critical for:

• Aerodynamics: Calculating lift and drag in aircraft or balloon design.
• Chemical Engineering: Designing reactors and gas transport systems.
• Environmental Science: Analyzing atmospheric gas distributions.

4. Using the Calculator

Example 1: Calculate the density of air at 1 atm and 20°C, with \( R = 8.31446261815324 \, \text{J/(mol·K)} \), output in kg/m³ and lb/cu ft:

• Gas: Air (\( M = 28.96 \, \text{g/mol} = 0.02896 \, \text{kg/mol} \))
• Pressure: \( P = 1 \, \text{atm} = 101325 \, \text{Pa} \)
• Temperature: \( T = 20 \, \text{°C} = 293.15 \, \text{K} \)
• Calculation: \( \rho = \frac{0.02896 \times 101325}{8.31446261815324 \times 293.15} \approx 1.20466 \, \text{kg/m³} \)
• Unit conversion: \( 1.20466 \times 0.062428 \approx 0.07520 \, \text{lb/cu ft} \)

Results:

• Gas Density: 1.20466 kg/m³
• Gas Density (converted): 0.07520 lb/cu ft

Example 2: Calculate the density of methane at 100 kPa and 77°F, output in g/L and g/cm³:

• Gas: Methane (\( M = 16.04 \, \text{g/mol} = 0.01604 \, \text{kg/mol} \))
• Pressure: \( P = 100 \, \text{kPa} = 100000 \, \text{Pa} \)
• Temperature: \( T = 77 \, \text{°F} = (77 - 32) \times \frac{5}{9} + 273.15 = 298.15 \, \text{K} \)
• Calculation: \( \rho = \frac{0.01604 \times 100000}{8.31446261815324 \times 298.15} \approx 0.64724 \, \text{kg/m³} = 0.64724 \, \text{g/L} \)
• Unit conversion: \( 0.64724 \times 0.001 \approx 0.00065 \, \text{g/cm³} \)

Results:

• Gas Density: 0.64724 g/L
• Gas Density (converted): 0.00065 g/cm³

5. Frequently Asked Questions (FAQ)

Q: What is gas density?
A: Gas density is the mass of a gas per unit volume, calculated using the ideal gas law, typically in kg/m³ or other units like g/L or lb/cu ft.

Q: How do I choose a gas?
A: Select a predefined gas (e.g., Air) or choose "Custom" to enter a molecular mass.

Q: Why is temperature in Kelvin required?
A: The ideal gas law uses absolute temperature in Kelvin to ensure positive values and accurate calculations.

Q: Does this work for real gases?
A: Yes, for low pressures and high temperatures where ideal gas assumptions apply. For high pressures, real gas models may be needed.

Q: Can I change the universal gas constant?
A: Yes, the default is 8.31446261815324 J/(mol·K), but you can edit it for custom calculations.

Gas Density Calculator © - All Rights Reserved 2025